Generating coherence-constrained multisensor signals using balanced mixing and spectrally smooth filters

Abstract

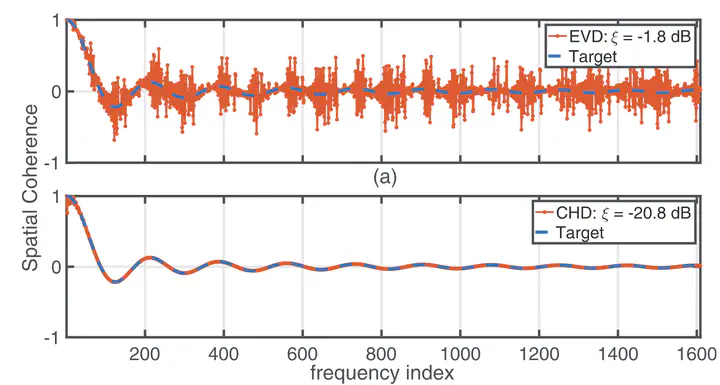
The spatial properties of a noise field can be described by a spatial coherence function. Synthetic multichannel noise signals exhibiting a specific spatial coherence can be generated by properly mixing a set of uncorrelated, possibly non-stationary, signals. The mixing matrix can be obtained by decomposing the spatial coherence matrix. As proposed in a widely used method, the factorization can be performed by means of a Choleski or an eigenvalue decomposition. In this work, the limitations of these two methods are discussed and addressed. In particular, specific properties of the mixing matrix are analyzed, namely the spectral smoothness and the mix balance. The first quantifies the mixing matrix-filters variation across frequency and the second quantifies the amount of input signals that contribute to each output signal. Three methods based on the unitary Procrustes solution are proposed to enhance the spectral smoothness, the mix balance and both properties jointly. A performance evaluation confirms the improvements of the mixing matrix in terms of objective measures. Further, the evaluation results show that the error between the target and the generated coherence is lowered by increasing the spectral smoothness of the mixing matrix.